Ergodicity: The Power of Systematic Diversification
Originally sent to clients of Upstart Wealth Management
In our last post, we explored risk at the individual level, drawing from ergodicity concepts to help. Specifically, we learned that:
Risk should be considered as a distribution of potential outcomes rather than as an average expected return.
Risk/Reward tradeoffs don’t happen in a vacuum; an individual’s circumstances must be considered before accepting a bet.
Risk is often experienced in series, rather than isolation. When risks stack together, the chance of failure can increase dramatically.
These concepts are especially relevant for the employee (or founder) of an early-stage tech company. If their company finds success, there’s often a significant personal windfall in the form of a liquidity event. At that time, consider:
The average investment return of a tech company’s stock doesn’t matter; what matters are the results of their specific company.
Each employee has their own financial situation separate from the concentrated position.
The longer an employee holds their company stock, the longer risks have the chance to stack, increasing the odds of a blow-up event.
Let’s take, as a hypothetical example, the case of Reid, an employee who experiences a liquidity event with Tech Company A. This is Reid’s first liquidity event and he has no other significant savings or investments. His financial net worth consists of a $1,000,000 position in company stock. How should he proceed? Let’s put some math to the risk concepts we’ve talked about so far.
Suppose the company stock has the following annual risk/return characteristics:
Expected return: 20%
Standard Deviation: 51%
We’re using this standard deviation as it represents the average standard deviation of a stock in the Russell 2000 Growth index, a reasonable proxy for US tech stocks. Now, let’s run a simulation where the single stock earns a random return somewhere on the normal distribution curve implied by the return and deviation numbers above. After 11 years, we can observe the final portfolio value of the trial. For example, here’s the results of one trial:
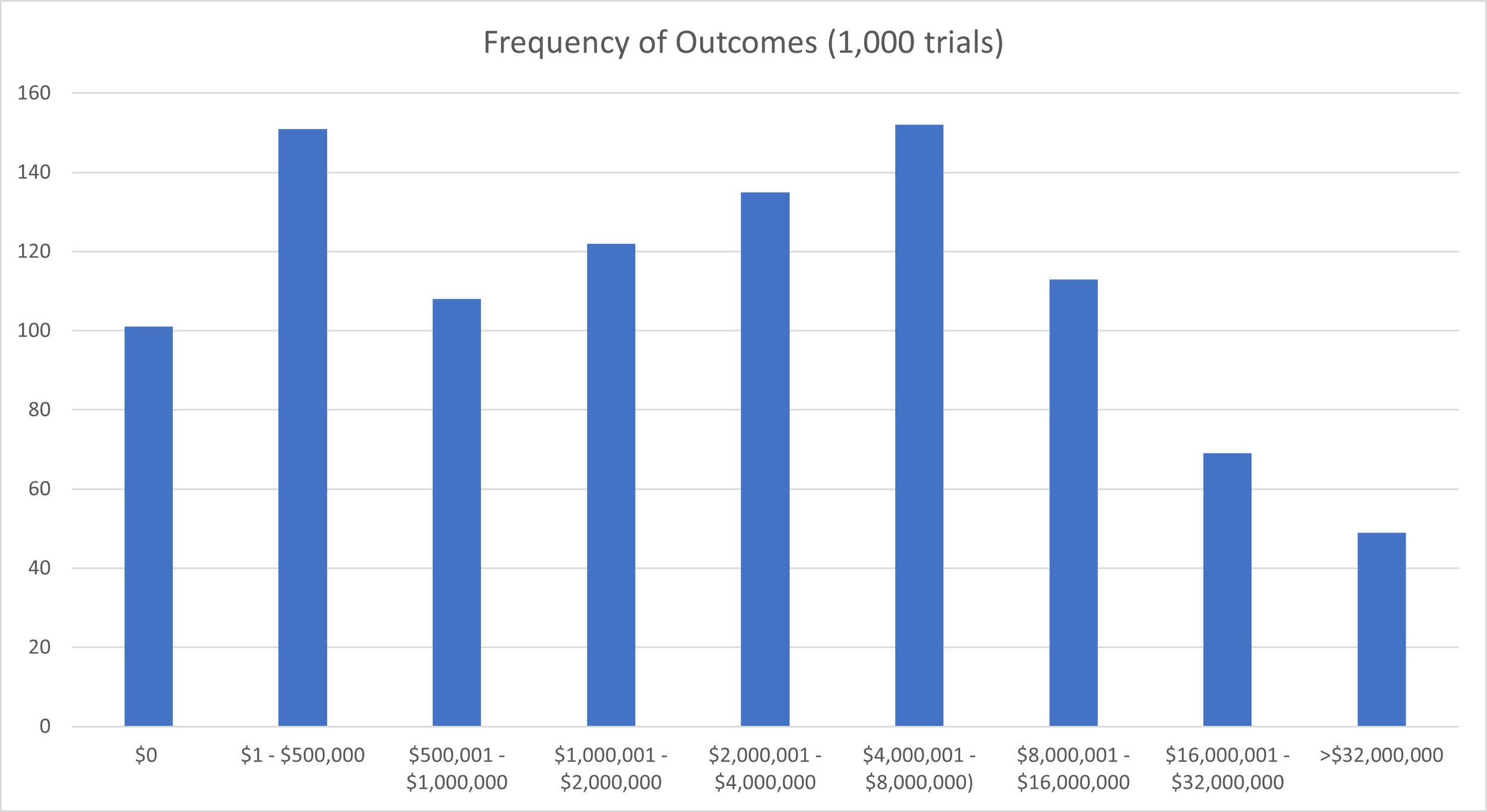
We can immediately see that the sequence of returns influences the final outcome (in this instance he goes for quite a ride but ends up right where he started), but it only shows us one of infinite outcomes that Reid could experience. We can’t build any decision rules based on one random iteration. Instead, let’s run 1,000 trials, observing his ending portfolio value in each instance (please note the scale is not linear):
It’s clear that, over time, a 20% annual expected return can lead to drastically disparate outcomes. There are a lot of simulations where he doubles, quadruples, or even octuples his money, and in roughly 12% of the simulations he ends up with over $16,000,000. However, he’s just as likely to end up with less than half of the original amount as he is to double it, and in 10% of the simulations he’s completely broke. Given his starting position, these downside risks may be considered unacceptable. The question is, how does he leave himself with plenty of upside exposure while significantly curtailing the downside risk?
Let’s consider an alternate strategy. At the beginning of each year, Reid plans to sell 15% of the shares he holds and reallocate those funds (after an assumed 24% tax haircut) to a globally diversified portfolio of publicly traded stocks with the following risk profile:
Expected Return: 7%
Standard Deviation: 16%
He does this regardless of the returns earned on the single stock - he’s selling 15% at the beginning of the year no matter what.
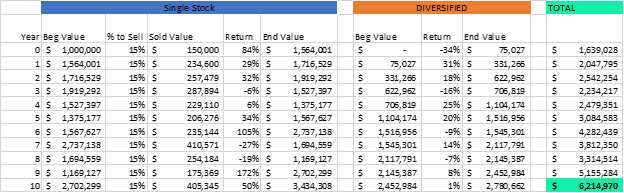
Again, here’s the results of a single simulation:
Now, let’s run this 1,000 times:
We can see that he’s much less likely to hit a grand slam, but he's much more likely to end with more than he started with. More importantly, there's only a single iteration out of 1,000 where he’s out of money. He’s successfully retained upside while substantially decreasing his risk of ruin. And while the focus here is on risk mitigation, locking in a baseline level of financial capital also grants Reid more latitude to take risks with his human capital in the future.
The idea of diversifying out of a position over time isn't new. But the ergodicity principles supporting a dollar-cost-averaging strategy out of a concentrated position might be. Diversifying adds resilience in the face of risk. The goal of this buffer isn't to remove all risk; we've already established that risk and return are inextricable. Rather, the buffer creates the ability to maintain risk exposure with portions of your financial or human capital. There can be a psychological burden that early employees feel when selling out of a company they worked hard to build. Reframing the diversification question as an opportunity to place other bets in the future can help alleviate that internal conflict and orient towards an abundant future.
Disclosure
The simulated returns portrayed above are purely for illustrative purposes and not representative of any particular stock or other type of security. The returns associated with any security will fluctuate in value over time, such that an investor’s original principal balance may increase or decrease in value. Past performance is no guarantee of future returns. Diversification, including the concentrated position diversification strategy portrayed herein, can neither assure a profit or prevent a loss.